The concept of Chaos in dynamical systems

When studying the time evolution of a system we observe a lot of different behaviours among its orbits. The ruling equations are often simple, but even so we might not be able to predict the future. Whenever that is possible we have regular motion (for instance periodic or quasi-periodic) which do not possess the properties associated with chaotic dynamics.

There is still no definite mathematically rigorous definition of chaos. A deeper knowledge of dynamical behaviour will be essential to determine its basic features. Up to now people have generally agreed on the existence of four fundamental characteristics of chaotic (deterministic) dynamical systems. These properties refer to the asymptotic behaviour of orbits inside the (invariant) region of phase space where chaos is believed to exist.

• Recurrence. There are always orbits which return an infinite number of times to an arbitrarly close neighbourhood of each point.
• Regularity. There is a lot of periodic orbits, in fact their union is dense.
• Unpredictability. Two initially arbitrarly close orbits will exponentially diverge with time.
• Universality. Despite the apparently 'unordely' behaviour we can compute numbers which are invariant under coordinate changes and families: Lyapunov exponents (to measure the exponentially divergence between orbits), entropy (a measure of chaos) and universal constants (e.g. the ratio between period doublings in universal parameter families) and self-similarity.

This is fundamentally different from stochastic systems or any kind of random systems.

Chaos is believed to appear when systems exhibit hyperbolic dynamics. Roughly, it means that orbits 'follow' some main directions which are exponentially contracting or expanding. If this happens similarly at every point the system is often labelled as uniformly hyperbolic. However, this turns out not to be the sole situation. In fact, most systems appearing in Nature do not seem to agree entirely with such a picture. Instead, orbits 'feel' a mixture of non-hyperbolic and hyperbolic behaviours, thus leading to more complicated dynamics.

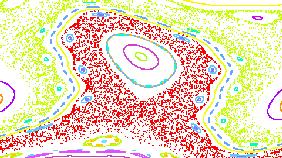
non-twist area-preserving map
regions of strong instability and "chaos"

João Lopes Dias
April 2005
Lisbon, Portugal